The video clips illustrating complex dynamics typically have a few MB and a duration of about 30s. They were produced with FFmpeg, combining images made with Mandel. For each video, two links are given: clicking play will open the video in a new browser tab or window. Or right-click to save the file to your hard disk, and open it with a video player. Clicking show opens a small pop-up box to play the video in reduced size.

Rational maps with a superattracting 2-cycle

Quadratic rational maps fb(z) = (z2 + b) / (1 - z2) form a family denoted by V2 , since the critical point ∞ is 2-periodic. The videos illustrate various applications of the Thurston algorithm to construct these maps from polynomials or from each other. The visualization of mating and anti-mating is based on the Buff–Chéritat approach to slow mating.

Most of the videos below give only six different maps, but up to four different constructions of the same map.
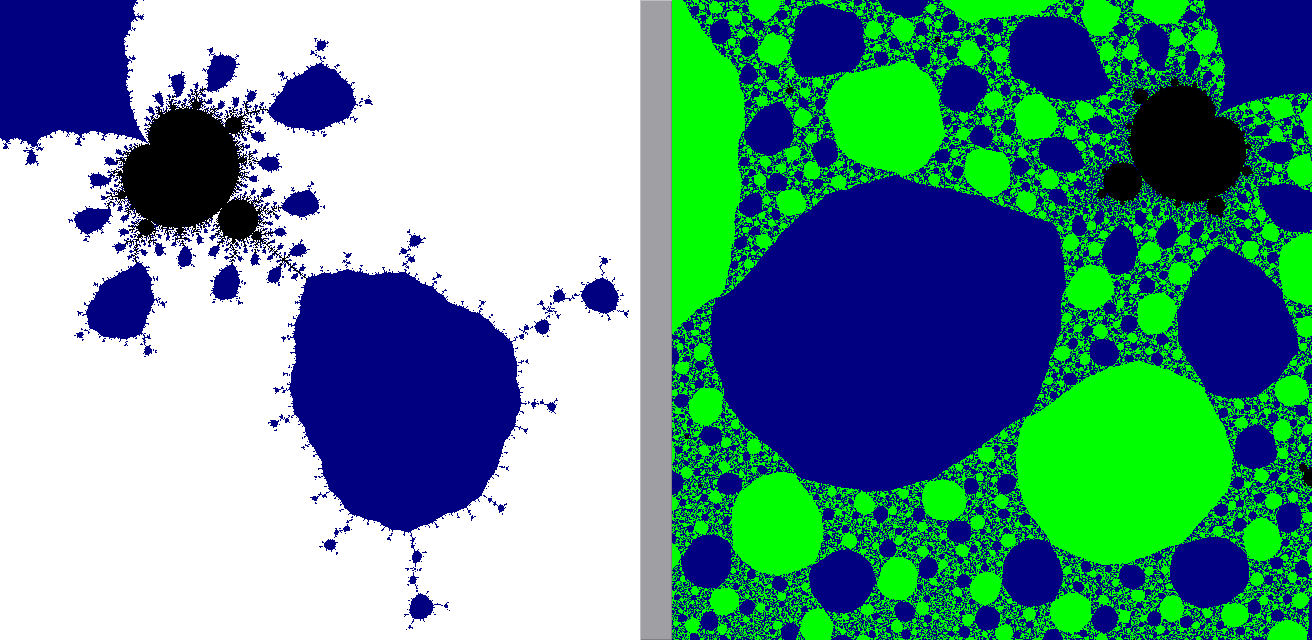
Here they are shown in parallel: play — show.
X

Left top: mating of z2 + i and z2 - 1.
Right top: anti-mating of z2 - q2 and z2 + q with q3 = √2 - 1.
Left bottom: repelling capture at the inverted Basilica.
Right bottom: regluing of a map of type C.

Mating and repelling capture

Two postcritically finite quadratic polynomials P(z) and Q(z) are combined to define the formal mating P ⊔ Q: in the lower and upper half-spheres, the new map is non-analytically conjugate to P(z) or Q(z), respectively. The Thurston algorithm gives a rational map, if P(z) and Q(z) do not belong to conjugate limbs of the Mandelbrot set. In particular, if Q(z) = z2 - 1 is the Basilica polynomial and P(z) does not belong to the 1/2-limb, the resulting map will be of the form fb(z) . It is conjugate to the topological mating P ∐ Q, where the filled Julia sets are glued together.

If P(z) is critically preperiodic, the same mating can be constructed by a repelling capture according to Rees: in the dynamic plane of Q(z), the critical value ∞ is moved to a preperiodic point along an external ray. The videos show this process inverted, that is, the Basilica contains ∞, and 0 is moved.

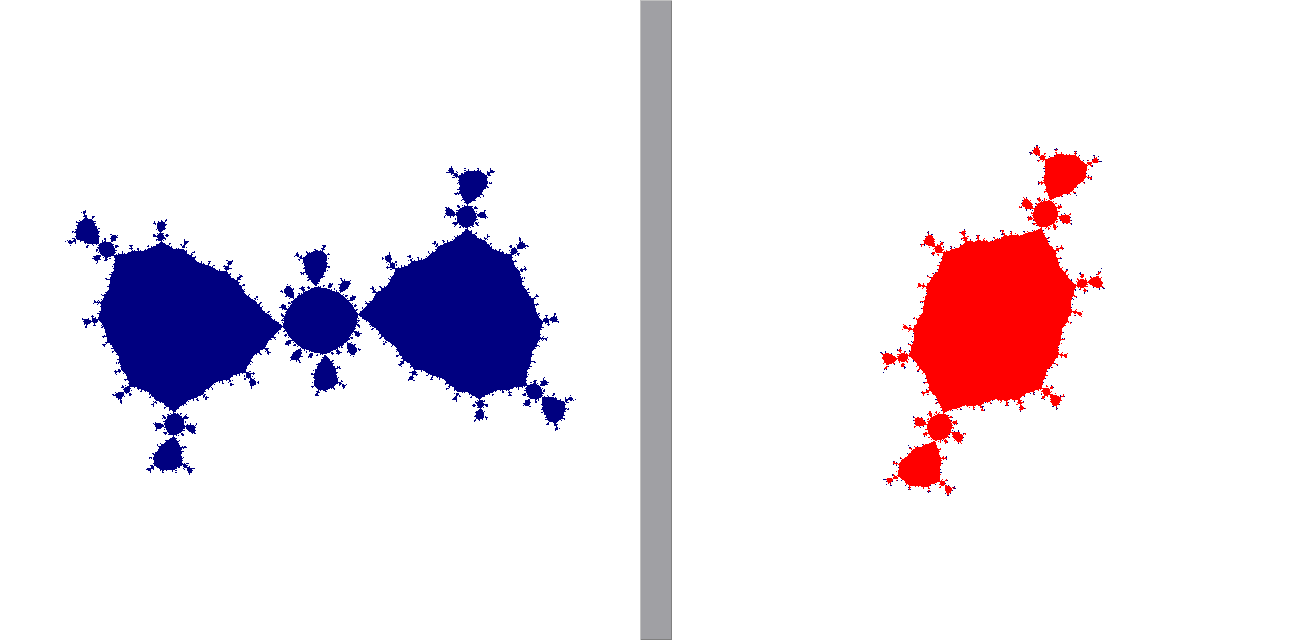
s3: the iconic mating of Rabbit and Basilica, displayed on the sphere: play — show.
X


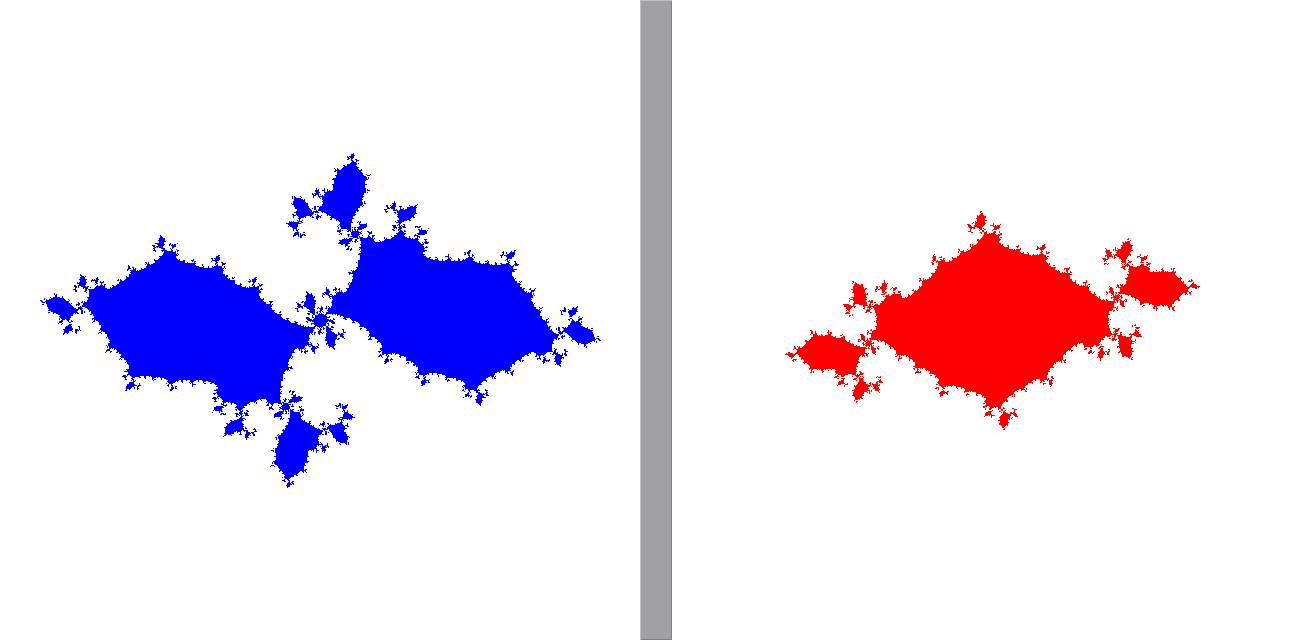
p4: the Kokopelli, a primitive period 4: play — show.
X


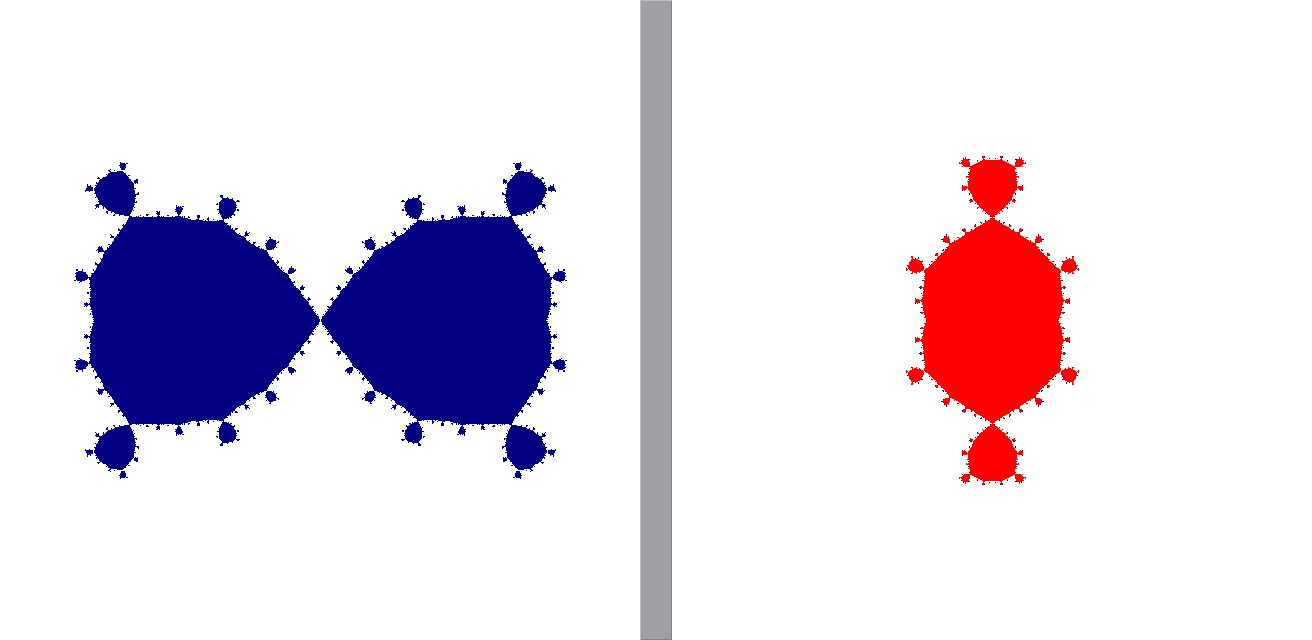
12: preperiod 1, period 2. The repelling 2-periodic points are distinct in the formal mating, but identified in the limit and in the topological mating: play — show.
X

The same mating as a repelling capture. The identification is immediate: play — show.
X


14: preperiod 1, period 4. The formal mating has no removable obstruction: play — show.
X

And the same mating as a repelling capture: play — show.
X


32: preperiod 3, period 2. A shared Basilica mating with 7/24 on the left and 5/24 on the right: play — show.
X


Jointly with Arnaud Chéritat: Since 349525/1048576 < 1/3 < 349526/1048576, the former mating with the Basilica exists, while the latter is obstructed; the composition of two iterates converges to the polynomial with parameter γ(1/1024): play — show.
X


Deformation in parameter space from M to V2 : play — show.
X


The locus of P in the 1/3-limb mated with the Basilica: play — show.
X


The locus of P on the 1/4-vein mated with the Basilica: play — show.
X

Anti-mating

Anti-mating was introduced by Ahmadi, Timorin, and Meyer. Consider two polynomials acting between two planes, P(z) = z2 + p from the first plane to the second plane, and Q(z) = z2 + q in the opposite direction. Mapping these planes to half-spheres defines the formal anti-mating P ⊓ Q. The topological anti-mating P ∏ Q is obtained by gluing the filled Julia sets of Q°P and P°Q along their boundaries. If p = -q2, the critical point at ∞ will be 2-periodic, so the anti-mating belongs to V2 . See
X
corresponding regions of the connectedness locus of (z2 - q2)2 + q and of V2 . While mating gives hyperbolic maps of type D only, anti-mating gives types C and B as well. See the forthcoming paper [B8].

p4: anti-mating gives the primitive period 4 (see Julia sets):
X
play — show.
X

And on the sphere, together with the mating above: play — show.
X


px: mating and anti-mating gives the primitive period 10 in the 4/9 limb (see Julia sets):
X
play — show.
X


12: anti-mating gives preperiod 1 and period 2, identified to 1 (see Julia sets):
X
play — show.
X


c4: anti-mating gives a hyperbolic capture of preperiod 4 (see Julia sets):
X
play — show.
X


Slow deformation of the quartic locus B in parameter space: play — show.
X

Hyperbolic capture and regluing

Starting with the Basilica polynomial, move the critical value ∞ to a preimage of the 2-periodic critical point 0, preferably along external and internal rays according to Wittner. Again, this process will be shown inverted, with 0 moved to the Basilica around ∞.

Both hyperbolic capture and repelling capture can be applied to postcritcally finite maps fb(z) in V2 , not only to the inverted Basilica. In particular, for a C component the center and the preperiodic maps on the boundary can be related by moving the critical value fb(0); this corresponds to the topological process of regluing.

c1: the critical value 0 is moved to a preimage of ∞ of order 1. The Julia set is symmetric under reflection, quarter-rotation, and inversion: play — show.
X


c4: the critical value 0 is moved to a preimage of ∞ of order 4. Note that the resulting rational map was obtained as an anti-mating as well: play — show.
X


12 as a regluing of c1: play — show.
X

c1 as a regluing of 12: play — show.
X


14 as a regluing of c1: play — show.
X

c1 as a regluing of 14: play — show.
X

Dehn twist

Applying a right Dehn twist to a mated Rabbit gives a mated Corabbit. (A left twist would give the obstructed mating of Airplane and Basilica). play — show (15 MB).
X